fractions decimals and percentages problem solving

Decimal, Percent and Fractions Practice Questions

• Posted by Brian Stocker MA
• Date March 27, 2014
• Comments 12 comments

Basic Math Practice

Basic math questions similar to those found on most high school exit or proficiency exam , nurse entrance tests

Percent Tips and Tricks

Practice Questions

1. 8 is what percent of 40?

a. 10% b. 15% c. 20% d. 25%

2. 9 is what percent of 36?

3. Three tenths of 90 equals:

a. 18 b. 45 c. 27 d. 36

4. .4% of 36 equals:

a. 1.44 b. .144 c. 14.4 d. 144

5. The ratio of 8:5 = (?)%

a. 75% b. 150% c. 175% d. 160%

6. 3/5 = (?)% x 5/8

a. 75% b. 100% c. 78% d. 96%

7. Express 2/5 as a decimal.

a. .33 b. .3 c. .4 d. .5

Try a FREE Math Quiz

8. Express .56 as percent.

a. 56% b. .56% c. 5.6% d. .056%

9. Express 18/6 as a percentage.

a. 300% b. 30% c. 150% d. 3%

10. Express 4/20 as a percentage.

a. 25% b. 20% c. 15% d. 40%

11. 7.5/30% = ?

a. 30 b. 35 c. 25 d. 20

12. Express .061 as percent.

a. .61% b. 6.1% c. 61% d. .061%

13. Express the ratio of 7:25 as percent.

a. 20% b. 22% c. 25% d. 28%

14. Express 7/16 as percent.

a. 43.75% b. 41.25% c. 42.5% d. 45%

1. C.  Finding the percentage of one number and another.

2. D.  Finding the percentage of one number and another.

3. C . Determining percent from a whole number.

4. B . Determining the fractional percentage of a number.

5. D . Determining the percentage of a ratio statement. The ratio of 8:5 must be converted to percent, or x/100, so 8/5 = x/100 – 800 = 5x – x = 160

6. D . Percentage of a fraction and another fraction. 3/5 = x/100 * 5/8 3/5 = 5x/800 25x = 2400 x = 96

7. C.  Converting common fraction to a decimal fraction.

8. A . Converting decimal fraction to a percentage.

9. A . Converting fraction to percent.

10. B . Converting fraction to percent.

11. C . Dividing a decimal fraction by a percentage.

12. B . Converting decimal fractions to percentages.

13. D . Converting a ratio to a percentage. Convert the ration 7:25 to percent, or x/100 – so 7/25 = x/100 + 700 = 25x = x = 28%

14. A . Converting a common fraction to a percentage.

Most Common Basic Math Mistakes

• , such as misreading a problem, simple arithmetic mistakes, or other careless errors
• Not showing all of the steps.  This makes it difficult for the teacher to understand how you got the answer.
• Not checking your work or not reviewing their test before turning it in.  Never leave the test room early!
• Not understanding the problem and solving it with an inappropriate method.
• Not understanding of basic math concepts and operations, such as fractions, decimals , and basic algebra .
• Not paying attention to the units of measure.
• Not understanding basic terminology, such as “factor,” “product,” and “quotient.”
• Not paying attention to the sign of the answer or confusing the sign.
• Not using the correct formula or equation for the problem.

Most Common Fraction Mistakes on a Test

1. Not simplifying fractions first.  Always simplify fractions to the simples form before adding, subtracting or other operations.

2. Not understanding common denominators.

To add or subtract fractions, they must have the same denominator. For example, to add 1/2 and 3/4, a common denominator is needed. The common denominator 4, because 4 is a multiple of both 2 and 4. So, you would convert 1/2 to 2/4 and add it to 3/4 to get 2/4 + 3/4 = 5/4

3. Errors with mixed numbers and converting to improper fractions or vice versa.

Referring to the problem above, 5/4 is an improper fraction, since 5 (the numerator) is larger than 4 (the denominator).  This can be converted to a mixed number – 5/4 = 4/4 + 1/4, and we know 4/4 = 1

so – 1 + 1/4 = 1 1/4.

4. Errors with equivalent fractions and reducing to the simplest form.

Here is question – Does 2/4 = 1/2 ?    YES!   we can reduce 2/4 by dividing the numerator (top)  and the denominator (bottom) by 2.  so 2/4 divided by 2/2 = 1/2.

5. Errors canceling common factors in fractions.

Cancelling out common factors works like this – 2/4 X 4/8    These are divisible by 2 so we divide by 2 in the top of one side and bottom of the other –  1/4 X 4/4

We can do the same again with the bottom of the first fraction and the top of the second – 1/1 X 1/4   and since 1/1 = 1  we have 1 X 1/4 = 1/4.

6. Errors with basic arithmetic operations (addition, subtraction, multiplication, and division) with fractions.  Fractions Practice

7. Solving word problems involving fractions.   How to Solve Word Problems   Word Problem Practice

8. Errors converting percent, decimals, and fractions. Converting Fractions and Decimal

Decimal Tips, Tricks and Shortcuts

Basic Math Multiple Choice

Converting Fractions to Decimals

Tag: Basic Math , Fractions , Percent Decimals , Practice Questions

Previous post

English Usage Practice Questions

Basic physics practice questions, you may also like.

Basic Math Video Tutorials

How to answer basic math multiple choice, how to solve linear inequalities – quick review and practice.

Basic linear inequalities have one of the following forms: ax + b > 0 ax + b < 0 ax + b > 0 ax + b < 0 where a and b are some real numbers. Our solution to …

12 Comments

.4% of 36 = .4/100 * 36 = (.4 *36)/100 = 14.4/100 = 0.144 The answer is correct

Thank you I so had it wrong

THIS WAS SO EASY

The question is .4% of 36

OOH!! I see the point now. that’s true you are correct sorry I don’t see the point before the 4

Sooooo……….How would I Know to do the last step(14.4/100) to complete the problem?

OK 14.4/100 – move decimal 2 places left – (dividing so the result will be smaller) = 0.144

Thank you Brian for working that one out, it was very helpful.

hi, can you please work out one of the ratio ones like #5 or #13

7:25= 7/25 7/25=0.28 0.28=28% Because from decimal to percent you move your decimal over two places to the right.

I got perfect score, thanks for this practice Ilove Math ♥

wow its very helpfull thank you :>>>> !i!i!i!i!

Leave A Reply Cancel reply

Your email address will not be published. Required fields are marked *

• Home   |  
• About   |  
• Contact Us   |  
• Privacy   |  
• Newsletter   |  
• Shop   |  
• 🔍 Search Site
• Christmas Worksheets
• Kindergarten
• All Generated Sheets
• Place Value Generated Sheets
• Addition Generated Sheets
• Subtraction Generated Sheets
• Multiplication Generated Sheets
• Division Generated Sheets
• Money Generated Sheets
• Negative Numbers Generated Sheets
• Fraction Generated Sheets
• Place Value Zones
• Number Bonds
• Addition & Subtraction
• Times Tables
• Fraction & Percent Zones
• All Calculators
• Fraction Calculators
• Percent calculators
• Area & Volume Calculators
• Age Calculator
• Height Calculator
• Roman Numeral Calculator
• Coloring Pages
• Fun Math Sheets
• Math Puzzles
• Mental Math Sheets
• Online Times Tables
• Online Addition & Subtraction
• Math Grab Packs
• All Math Quizzes
• Kindergarten Math Quizzes
• 1st Grade Quizzes
• 2nd Grade Quizzes
• 3rd Grade Quizzes
• 4th Grade Quizzes
• 5th Grade Quizzes
• 6th Grade Math Quizzes
• Place Value
• Rounding Numbers
• Comparing Numbers
• Number Lines
• Prime Numbers
• Negative Numbers
• Roman Numerals
• Subtraction
• Add & Subtract
• Multiplication
• Fraction Worksheets
• Learning Fractions
• Fraction Printables
• Percent Worksheets & Help
• All Geometry
• 2d Shapes Worksheets
• 3d Shapes Worksheets
• Shape Properties
• Geometry Cheat Sheets
• Printable Shapes
• Coordinates
• Measurement
• Math Conversion
• Statistics Worksheets
• Bar Graph Worksheets
• Venn Diagrams
• All Word Problems
• Finding all possibilities
• Logic Problems
• Ratio Word Problems
• All UK Maths Sheets
• Year 1 Maths Worksheets
• Year 2 Maths Worksheets
• Year 3 Maths Worksheets
• Year 4 Maths Worksheets
• Year 5 Maths Worksheets
• Year 6 Maths Worksheets
• All AU Maths Sheets
• Kindergarten Maths Australia
• Year 1 Maths Australia
• Year 2 Maths Australia
• Year 3 Maths Australia
• Year 4 Maths Australia
• Year 5 Maths Australia
• Meet the Sallies
• Certificates

Fractions Decimals Percents Worksheets

Welcome to our Fractions Decimals Percents Worksheets page. Here you will find a wide range of printable Fraction Worksheets which will help your child understand and practice how to convert between fractions, decimals and percentages.

Looking to convert fractions to percentages or decimals; decimals to fractions or percentages; percentages to fractions or decimals?

Then look no further - we have what you need!

You can also try our NEW quick quiz at the bottom of this page to test your skills online.

For full functionality of this site it is necessary to enable JavaScript.

Here are the instructions how to enable JavaScript in your web browser .

Quicklinks to ...

• Fractions Decimals Percents Conversion Table

Fractions Decimals Percents Riddles

• More recommended resources

Fractions Decimals Percents Online Quiz

Fractions decimals percents.

Below are some common conversions for fractions into decimals and percents.

Where a digit is underlined, it means that the number has been rounded to 3 decimal places, or to the nearest 0.1%.

We have split up our fractions decimals percents worksheets into several different sections to make it easier for you to choose the skill you want to practice.

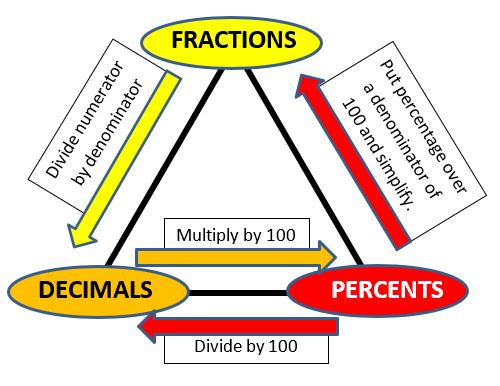
• The first section is just converting fractions into decimals and percents.
• The second sections is about converting decimals to percents and fractions.
• The third section covers convertig percents to fractions and decimals.
• The last section involves converting between all three.

The sheets are carefully graded so that the supported and easier sheets come first, and the most difficult sheet is the last one.

Using these sheets will help your child to:

• convert between fractions decimals and percents.

These sheets are aimed at 5th, 6th and 7th graders.

Converting Fractions to Decimals and Percents

• Fractions to Decimals and Percents Sheet 1
• PDF version
• Fractions to Decimals and Percents Sheet 2

Fractions to Decimals and Percents Walkthrough Video

This short video walkthrough shows several problems from our Fractions to Decimals and Percents Worksheet 1 being solved and has been produced by the West Explains Best math channel.

If you would like some support in solving the problems on these sheets, please check out the video below!

Converting Decimals to Percents and Fractions

• Decimals to Percents and Fractions Sheet 1
• Decimals to Percents and Fractions Sheet 2

Converting Percents to Decimals and Fraction s

• Percents to Decimals and Fractions Sheet 1
• Percents to Decimals and Fractions Sheet 2

Convert Between Fractions Decimals Percents Worksheets

• Fractions Decimals and Percents Sheet 1
• Fractions Decimals and Percents Sheet 2
• Fractions Decimals and Percents Sheet 3
• Fractions Decimals and Percents Sheet 4

Now is your chance to practice your fractions decimals and percents problem solving skills with some fun riddles!

• Fraction Decimal Percent Riddle 1
• Fraction Decimal Percent Riddle 2
• Fraction Decimal Percent Riddle 3

More Recommended Math Worksheets

Take a look at some more of our worksheets similar to these.

Equivalent Fractions

The printable fraction page below contains support, examples and practice using equivalent fractions.

• Finding Equivalent Fractions support page
• Equivalent Fractions Worksheets

Comparing Fractions

We have some carefully graded worksheets on comparing and ordering fractions.

You can choose from supported sheets with diagrams for students who need extra help to harder worksheets for those more confident.

• Comparing Fractions Worksheet page

Simplifying Fractions

Take a look at our Simplifying Fractions Practice Zone or try our worksheets for finding the simplest form for a range of fractions.

You can choose from proper fractions, improper fractions or both.

You can print out your results or benchmark your scores against future achievements.

Good for practising equivalent fractions as well as converting to simplest form.

Great for using with a group of children as well as individually.

• Simplify Fractions Practice Zone
• Simplifying Fractions Worksheet page

Fraction Riddles

Riddles are a great way to get children to apply their knowledge of fractions.

These riddles are a good way to start off a maths lesson, or also to use as a way of checking your child's understanding about fractions.

All the fraction riddles consist of 3 or 4 clues and a selection of 6 or 8 possible answers. Children have to read the clues and work out which is the correct answer.

The riddles can also be used as a template for the children to write their own clues for a partner to guess.

• Fraction Riddles for kids (easier)
• Free Printable Fraction Riddles (harder)

Are you looking for free fraction help or fraction support?

Here you will find a range of fraction help on a variety of fraction topics, from simplest form to converting fractions.

There are fraction videos, worked examples and practice fraction worksheets.

Our quizzes have been created using Google Forms.

At the end of the quiz, you will get the chance to see your results by clicking 'See Score'.

This will take you to a new webpage where your results will be shown. You can print a copy of your results from this page, either as a pdf or as a paper copy.

For incorrect responses, we have added some helpful learning points to explain which answer was correct and why.

We do not collect any personal data from our quizzes, except in the 'First Name' and 'Group/Class' fields which are both optional and only used for teachers to identify students within their educational setting.

We also collect the results from the quizzes which we use to help us to develop our resources and give us insight into future resources to create.

For more information on the information we collect, please take a look at our Privacy Policy

We would be grateful for any feedback on our quizzes, please let us know using our Contact Us link, or use the Facebook Comments form at the bottom of the page.

This quick quiz tests your knowledge and skill at converting between fractions, decimals and percents.

Fun Quiz Facts

• This quiz was attempted 1,155 times in the last academic year.
• The average (mean) score was 13.4 out of 20 marks.
• Can you beat the mean score?

How to Print or Save these sheets 🖶

Need help with printing or saving? Follow these 3 steps to get your worksheets printed perfectly!

• How to Print support

Subscribe to Math Salamanders News

Sign up for our newsletter to get free math support delivered to your inbox each month. Plus, get a seasonal math grab pack included for free!

• Newsletter Signup

Return to 5th Grade Math Worksheets

Return to Fraction Worksheets

Return from Fractions Decimals Percents Worksheets to Math Salamanders Homepage

Math-Salamanders.com

The Math Salamanders hope you enjoy using these free printable Math worksheets and all our other Math games and resources.

We welcome any comments about our site or worksheets on the Facebook comments box at the bottom of every page.

New! Comments

TOP OF PAGE

© 2010-2024 Math Salamanders Limited. All Rights Reserved.

• Privacy Policy
• Copyright Policy

Advent Calendars 2024 Primary | Secondary

Or search by topic

Number and algebra.

• Properties of numbers
• Place value and the number system
• Calculations and numerical methods
• Fractions, decimals, percentages, ratio and proportion
• Patterns, sequences and structure
• Coordinates, functions and graphs
• Algebraic expressions, equations and formulae

Geometry and measure

• Measuring and calculating with units
• Angles, polygons, and geometrical proof
• 3D geometry, shape and space
• Transformations and constructions
• Pythagoras and trigonometry
• Vectors and matrices

Probability and statistics

• Handling, processing and representing data
• Probability

Working mathematically

• Thinking mathematically
• Mathematical mindsets

Advanced mathematics

• Decision mathematics and combinatorics
• Advanced probability and statistics

For younger learners

• Early years foundation stage

Fractions, Decimals and Percentages - Short Problems

This is part of our collection of Short Problems . You may also be interested in our longer problems on Fractions, Decimals and Percentages . Printable worksheets containing selections of these problems are available here .

Jacob's flock

Bouncing ball

Information display

Mean sequence

Better spelling

Valuable percentages

Ordering fractions

Multiplication magic square

Farthest fraction

Tommy's tankard

Smashing time

Talulah's tulips

Percentage mad

Smallest fraction

Charlie's money.

Pride of place

Between a sixth and a twelfth.

Magical products

Three blind mice

Second half score

Peanut harvest

Tricky fractions

Slightly outnumbered

Too close to call

Tennis club

The Grand Old Duke of York

What percentage of his 10,000 men did the Grand Old Duke of York have left when he arrived back at the bottom of the hill?

How many rats did the Pied Piper catch?

Entrance exam

Meeting point

Petrol station

A drink of water

Test scores

Percentage of a quarter

Percentage swap

Recurring mean

Pineapple juice.

Fractions of 1000

2011 digits

Percentage unchanged

Breakfast time

Itchy's fleas

Antiques roadshow

Elephants and geese

The property market

Producing an integer

Squeezed in

PROBLEM SOLVING WITH FRACTIONS DECIMALS AND PERCENTAGES WORKSHEET

Problems with fractions.

(1)  A fruit merchant bought mangoes in bulk. He sold 5/8  of the mangoes. 1/16 of the mangoes were spoiled. 300 mangoes remained with him. How many mangoes did he buy? 

(2)  A family requires 2 1/2 liters of milk per day. How much milk would family require in a month of 31 days?  

(3)  A ream of paper weighs 12 1/2 kg.  What is the weight per quire ?

(4)   It was Richard's birthday. He distributed 6 kg of candies to his friends. If he had given 1/8  kg of candies to each friend, how many friends were there ?

(5)  Rachel bought a pizza and ate 2/5 of it. If he had given 2/3 of the remaining to his friend, what fraction of the original pizza will be remaining now ?

Answer Key :

(1)  960 mangoes

(2)   77 1/2 liter

(3)  5/8 kg

(4)   48 friends

(5)  1/5

Fraction Word Problems Mixed Operations

(1)  Linda walked 2 1/3 miles on the first day and 3 2/5   miles on the next day. How many miles did she walk in all ?                Solution

(2)   David ate 2 1/7 pizzas and he gave 1 3/14    pizzas to his mother. How many pizzas did David have initially ?

(3)   Mr. A has 3 2/3 acres of land. He gave 1 1/4 acres of land to his friend. How many acres of land does Mr. A have now ?          Solution

(4)   Lily added 3 1/3 cups of walnuts to a batch of trail mix. Later she added 1 1/3 cups of almonds. How many cups of nuts did Lily put in the trail mix in all? 

(5)   In the first hockey games of the year, Rodayo played 1 1/2 periods and 1 3/4 periods. How many periods in all did he play ?         Solution

(6)   A bag can hold 1 1/2 pounds of flour. If Mimi has 7 1/2 pounds of flour, then how many bags of flour can Mimi make ?        Solution

(7)   Jack and John went fishing Jack caught 3 3/4 kg of fish and while John  caught 2 1/5 kg of fish. What is the total weight of the fish they caught?

(8)   Amy has 3 1/2 bottles in her refrigerator. She used 3/5 bottle in the morning 1 1/4 bottle in the afternoon. How many bottles of milk does Amy have left over ?  

(9)   A tank has 82 3/4 liters of water. 24 4/5 liters of water were used and the tank was filled with another 18 3/4 liters. What is the final volume of the water in the tank ?

(10)   A trader prepared 21 1/2 liters of lemonade. At the end of the day he had 2 5/8 liters left over. How many liters of lemonade was sold by the Trader? 

Answer key :

Problems on Decimals

(1)  A chemist mixed 6.35 grams of one compound with 2.45 grams of another compound. How many grams were there in the mixture.      Solution

(2)   If the cost of a pen is $10.50, a book is $25.75 and a bag is $45.50, the  find the total cost of 2 books, 3 pens and 1 bag.         Solution

(3)    John wants to buy a bicycle that cost $ 450.75. He has saved $ 125.35. How much more money must John save in order to have enough money to buy the bicycle ?

(4)   Jennifer bought 6.5 kg of sugar. she used 3750 grams. How many kilograms of sugar were left ?

(5)   The inner radius of a pipe is 12.625 mm and the outer radius is 18.025 mm. Find the thickness of the pipe.          Solution

(6)   A copy of English book weighs 0.45 kg. What is the weight of 20 copies ?          Solution

(7)   Find the weight of 25.5 meters of copper wire in kilograms, if one meter weighs 10 grams.          Solution

(8)   Robert paid $140 for 2.8 kg of cooking oil. How much did 1 kg of the cooking oil cost ?         Solution

(9)   If $20.70 is earned in 6 hours, how much money will be earned in 5 hours ?            Solution

(10)   A pipe is 76.8 meters long. What will the greatest number of pieces of pipe each 8 meters long that can be cut from this pipe ?          Solution

Answers Key :

Problems on Percentage

(1)  In a particular store the number of TV's sold the week of Black Friday was 685. The number of TVs sold the following week was 500. TV sales the week following Black Friday were what percent less than TV sales the week of Black Friday ?

(A)  17%   (B)  27%   (C)  37%   (D)  47%

(2)  In March, a city zoo attracted 32000 visitors to its polar bear exhibit. In April, the number of visitors to the exhibit increased by 15%. How many visitors did the zoo attract to its polar bear exhibit in April ?

(A)  32150   (B)  32480   (C)  35200  (D)  36800

(3)  A charity organization collected 2140 donations last month. With the help of 50 additional volunteers, the organization collected 2690 donations this month. To the nearest tenth of a percent, what was the percent increase in the number of donations the charity organization collected ?

(A) 20.4%   (B)  20.7%    (C)  25.4%   (D)  25.7%

(4)  The discount price of a book is 20% less than the retail price. James manages to purchase the book at 30% off the discount price at the special book sale. What percent of the retail price did James pay ?

(A)  42%   (B)  48%    (C)  50%   (D)  56%

(5)  Each day, Robert eats 40% of the pistachios left in his jar at the time. At the end of the second day, 27 pistachios remain. How many pistachios were in the jar at the start of the first day ?

(A)  75   (B)  80   (C)  85  (D)  95

(6) Joanne bought a doll at a 10 percent discount off the original price of $105.82. However, she had to pay a sales tax of x% on the discounted price. If the total amount she paid for the doll was $100, what is the value of x ?

(A)  2   (B)  3   (C)  4  (D)  5

(7)  In 2010, the number of houses built in Town A was 25 percent greater than the number of houses built in Town B. If 70 houses were built in Town A during 2010, how many were built in Town B ?

(A)  56   (B)  50    (C)  48   (D)  20

(8)  Over two week span, John ate 20 pounds of chicken wings and 15 pounds of hot dogs. Kyle ate 20 percent more chicken wings and 40 percent more hot dogs. Considering only chicken wings and hot dogs, Kyle ate approximately x percent more food, by weight, than John, what is x (rounded to the nearest percent) ?

(A)  25   (B)  27    (C)  29   (D)  30

(9) Due to deforestation, researchers, expect the deer population to decline by 6 percent every year. If the current deer population is 12000, what is the approximate expected population size in 10 years from now ?

(A)  25000   (B)  48000    (C)  56000   (D)  30000

(10)  In 2000 the price of a house was $72600. By 2010 the price of the house has increased to 125598.

(A)  70%    (B)  62%    (C)  73%    (D)  90%

Kindly mail your feedback to   [email protected]

We always appreciate your feedback.

© All rights reserved. onlinemath4all.com

• Sat Math Practice
• SAT Math Worksheets
• PEMDAS Rule
• BODMAS rule
• GEMDAS Order of Operations
• Math Calculators
• Transformations of Functions
• Order of rotational symmetry
• Lines of symmetry
• Compound Angles
• Quantitative Aptitude Tricks
• Trigonometric ratio table
• Word Problems
• Times Table Shortcuts
• 10th CBSE solution
• PSAT Math Preparation
• Privacy Policy
• Laws of Exponents

Recent Articles

SAT Math Resources (Videos, Concepts, Worksheets and More)

Dec 20, 24 06:25 AM

Digital SAT Math Problems and Solutions (Part - 89)

Dec 20, 24 06:23 AM

Solving Trigonometric Equations Problems and Solutions

Dec 18, 24 10:53 AM